Why Π Is In The Normal Distribution (Beyond Integral Tricks)

Exploring the Unexpected Applications of Mathematical Concepts

In Eugene Wigner's thought-provoking paper titled "The Unreasonable Effectiveness of Mathematics in the Natural Sciences," he shares an intriguing anecdote about two friends, one of whom is a statistician. When the statistician explains how pi is used in a population distribution formula, his friend is left astounded. This anecdote sets the stage for Wigner's exploration of the phenomenon where mathematical concepts find unexpected applications in the natural sciences.

The Mystery of Pi in the Formula for a Normal Distribution

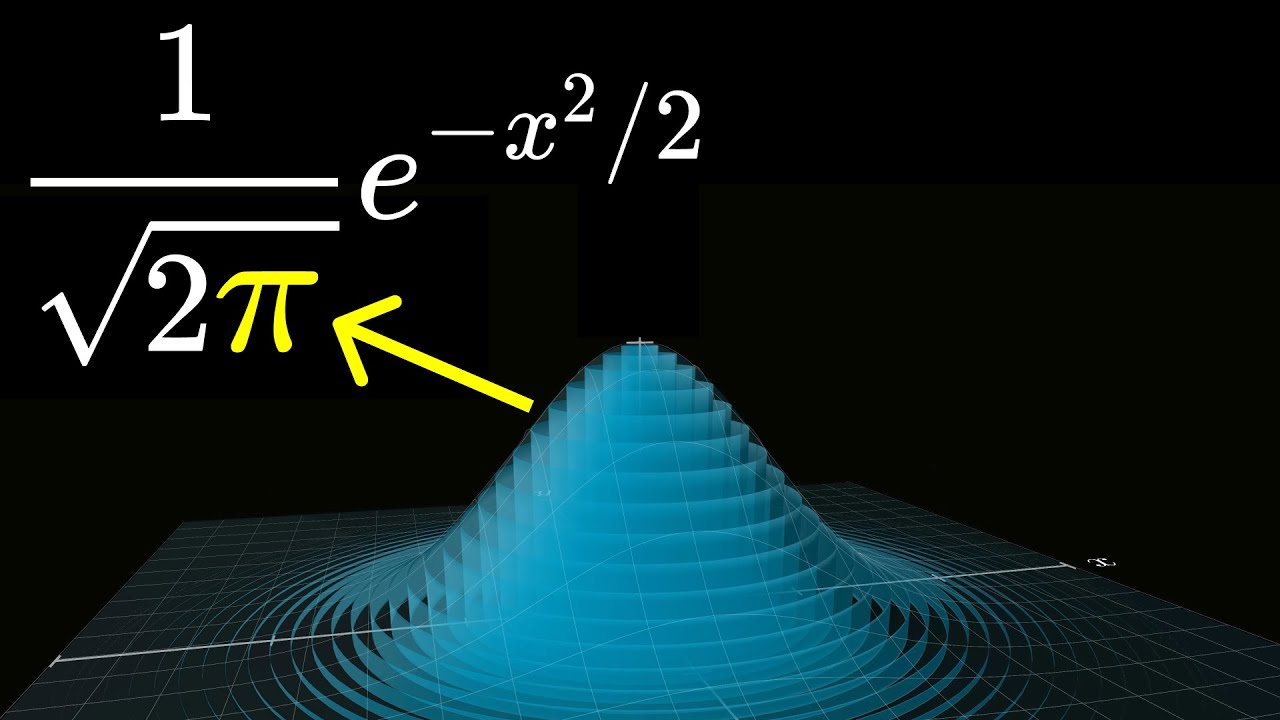
One particular focus of Wigner's paper is to unravel the presence of pi in the formula for a normal distribution. To understand this, we delve into the concept of finding the area under the curve described by the function e^-x^2. Typically, an integral is used to calculate this area. However, when dealing with the specific function e^-x^2, things become more challenging as it lacks a simple antiderivative.

A Clever Trick and the Central Limit Theorem

To overcome this challenge, a clever trick comes into play. This trick ultimately connects the presence of pi in the formula to what is known as the Central Limit Theorem. The Central Limit Theorem explains when a normal distribution arises in nature. By utilizing this theorem, mathematicians are able to calculate the area under the curve described by e^-x^2 and uncover its connection to pi.

Deriving the Gaussian Distribution: From Properties to Circular Symmetry

Moving on from Wigner's paper, let's explore how mathematicians derive the Gaussian distribution, also known as the normal distribution. It all begins with examining the probability density function for a point in two dimensions and recognizing that it must possess certain properties. These properties lead to a functional equation that needs to be solved.

To solve this equation, a helper function called h(x) is introduced. Through careful analysis, it becomes evident that h(x) must be an exponential function. This realization leads to the conclusion that the target function, f, takes the form e^cx^2. However, to ensure that this function is a valid probability distribution, the constant in the exponent must be negative. This constant determines the spread of the distribution.

The derivation of the Gaussian distribution is attributed to Herschel and later independently discovered by James Clerk Maxwell in three dimensions. The appearance of pi in this distribution can be explained by its